 CS 660: Combinatorial Algorithms
CS 660: Combinatorial Algorithms
Splay Tree
[To Lecture Notes Index]
San Diego State University -- This page last updated Sept 27, 1995

Contents of Splay Tree Lecture
- Self-Organizing BST
- Splay Trees
- Performance of Splay Tree
- Splay Operations
- Top-Down Splay
Basic Rotation

Simple Exchange (Transpose)
- When we access a node, apply a rotation to move the node one level closer
to root
-
- If each node is accessed with probability of 1/n the average search time is:


Move-to-root
- When we access a node, apply series of rotations to make the node the root
-
- We have a list of n items: a1, a2, ..., an
- Probability of accessing item ak is P(ak)
-
- The average search cost is[1]
-
-

-
- Where H(P(a1), ....P(an)) is the entropy of the distribution
-
- If P(ak) = 1/n then
-
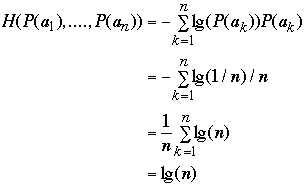
Move-to-root example

Splaying
Splay step at x
let p(x) = parent of node x
case 1 (zig) p(x) = root of the tree

case 2 (zig-zig) p(x) is not the root and x and p(x) are both left
(right) children
 case 3 (zig-zag) p(x) is not the root and x is a left (right) child and
p(x) is a right(left ) child
case 3 (zig-zag) p(x) is not the root and x is a left (right) child and
p(x) is a right(left ) child

To Splay a node X, repeat the splay step on X until it is the root
Splay B

Splay vs. Move-to-root
Case 1

Case 2
 Splay vs. Move-to-root
Splay vs. Move-to-root
Case 3

Move-to-root A

Splay A

Splaying at a node of depth d takes Theta(d) time
ck= actual cost of operation k
 =
amortized cost of operation k
=
amortized cost of operation k
Dk = the state of the data structure after applying k'th operation to Dk
 = potential associated with Dk
= potential associated with Dk
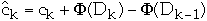
so we get:
-

-
-
The actual amount of work required is given by:
-

So need the total amortized work and difference in potential
Potential for Splay Trees
Let:
- w(x) = weight of node x, a fixed but arbitrary value
-
- size(x) =

-
- rank(x) = lg(size(x))
-
-

Example
Let w(x) = 1/n where n is the number of nodes in the tree
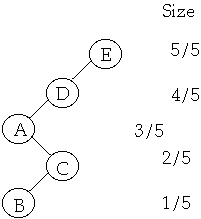
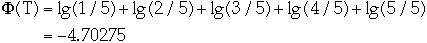
Lemma The amortized time to splay node x in a tree with root at t is at
most 3(r(t) - r(x)) + 1 = O(lg(s(t)/s(x)))
- Let s, r denote the size, rank functions before a splay
-
- Let s', r' denote the size, rank functions after a splay
-
- Count rotations
Case 1 (zig) One rotation

Amortized time of this step is:
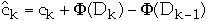
1 + [r'(x) + r'(y)] - r(x) - r(y) only x and y change rank
- <= 1 + r'(x) - r(x)
- r(y) >= r'(y)
-
- <= 1 + 3(r'(x) - r(x))
- r'(x) >= r(x)
Case 2 (zig-zig) Two rotations

Amortized time of this step is:
2 + r'(x) + r'(y) + r'(z)
- r(x) - r(y) - r(z) only x, y ,z change rank
- = 2 + r'(y) + r'(z) - r(x) - r(y)
- r'(x) = r(z)
-
- <= 2 + r'(x) + r'(z) - 2r(x)
- r'(x) >= r'(y) and
-
- r(y) >= r(x)
Assume that 2r'(x) - r(x) - r'(z) >= 2
2 + r'(x) + r'(z) - 2r(x)
- <= 2r'(x) - r(x) - r'(z) + r'(x) + r'(z) - 2r(x)
-
- = 3r'(x) - 3r(x)
-
Need to show 2r'(x) - r(x) - r'(z) >= 2
Claim 1
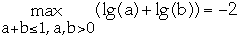
- Set b = 1-a
-
- We have

-
- Setting this to 0 to find extreme value we get
-
-

-
- so
-
-

-
- that is a = 1/2 and b = 1/2
-
- but lg(1/2)+lg(1/2)= -2
End claim 1
Claim 2 2r'(x) - r(x) - r'(z) >= 2
- Recall that:

-
-
- We have:
- r(x) + r'(z) - 2r'(x) = lg(s(x)) + lg(s'(z)) - 2lg(s'(x))
-
-
- = lg(s(x)/s'(x)) + lg(s'(z)/s'(x))
-
- Now s(x) + s'(z) <= s'(x)
- (Why?)
-
- so
- 0<= s(x)/s'(x) + s'(z)/s'(x) <= 1
-
- Set s(x)/s'(x) = a and s'(z)/s'(x) =b in claim 1 to get
-
-
- lg(s(x)/s'(x)) + lg(s'(z)/s'(x)) <= -2
-
Thus r(x) + r'(z) - 2r'(x) <= -2 or 2r'(x) - r(x) - r'(z) >= 2Case
3 (zig-zag)

Amortized time of this step is:
2 + r'(x) + r'(w) + r'(z)
- r(x) - r(w) - r(z)
- <= 2 + r'(w) + r'(z) - 2r(x)
- r'(x) = r(z) and
-
- r(w) >= r(x)
Assume that 2r'(x) - r'(w) - r'(z) >= 2
2 + r'(w) + r'(z) - 2r(x) <= [2r'(x) - r'(w) - r'(z)] + r'(w) + r'(z) -
2r(x)
= 2r'(x) - 2r(x) <= 3 * ( r'(x) - r(x) )
Claim 3: 2r'(x) - r'(w) - r'(z) >= 2
- Proof: see claims 1 & 2
Putting it All together
Lemma The amortized time to splay node x in a tree with root at t is at
most 3(r(t) - r(x)) + 1 = O(lg(s(t)/s(x)))
Splay at B

Cost of Step 1 <= 3* ( r'(B) - r(B) ) case 3
Cost of Step 2 <= 3 * ( r''(B) - r'(B) ) case 2
Total cost = 3* ( r'(B) - r(B) ) + 3 * ( r''(B) - r'(B) )
- = 3 * ( r''(B) - r(B) )
-
- = 3 * ( r(E) - r(B) )
Case 1 only happens when splaying a child of the root
This happens at most once per splay
Amortized Cost of M Splay Operations
on Tree with N nodes
Let node i be accessed q(i) times.
Then
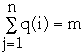
Theorem (Balance Theorem) The total access time is
- O( (m + n) * lg (n + m) )
Theorem (Static Optimality) If every item is accessed at least once,
then the total access time is:
-

Example
Let q( i ) = 1 then we have
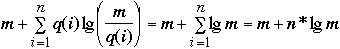
proof of Static Optimality:
Recall that:
- size(x) =

-
- rank(x) = lg(size(x))
-
-
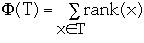
let w(i) = q(i)/m then
-
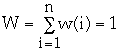
We wish to compute the actual cost of m operations.
Recall:
-
-

So we need to compute the change in potential and the amortized cost over m
operations.
First the change in potential.
The biggest change in potential comes when a node moves from root to a leaf.
Assume that all nodes start at the root and end up as a leaf.
This will give us an upper bound on the change in potential.
We have:
- rank of the root = lg(W)
-
- rank of node i as a leaf = lg( w( i ) )
So change in rank of node i is at most
- lg(W) - lg( w( i ) ) = lg( W/w(i) )
so the upper bound on the net decrease in potential over m operations is:
-

The amortized access time of item i is:
-

Amortized access of all times is
-
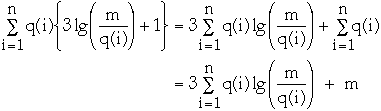
So the total cost is bounded by:
-
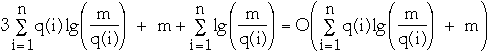
access(i, t): if i is in tree t return pointer to i, otherwise return null
pointer
- Find i, then splay tree t at i.
- If i is not in tree t, then splay last node accessed looking for i
join (a, b): Return tree formed by combining tree "a", and tree "b". Assumes
that every item in "a" has key less then every item in "b"
- Splay largest item in "a", then add "b" as a right child of root of "a"
split (i, t): Split tree t, containing item i, into two trees: "a",
containing all items with key less or equal to "i"; and "b", containing all
items with key greater than "i"
- Perform access(i, t) then split tree at root
insert(i, t): insert i in tree t
- Perform split (i, t) then make i the root of the two trees returned by
split
delete(i, t): delete i from tree t
- Perform access(i, t) then perform join on t's subtrees
Balance Theorem with Updates
A sequence of m arbitrary operations on a collection of initially empty splay
trees takes
 time, where nj is the number of items in the tree or trees involved in
operation j.
time, where nj is the number of items in the tree or trees involved in
operation j.
Case 1 X is the node we are splaying
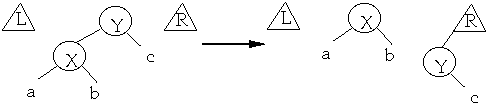
Case 2 (zig-zig) The node we are splaying is in the subtree rooted at
X
 Case
3 (zig-zag) The node we are splaying is in the subtree rooted at X
Case
3 (zig-zag) The node we are splaying is in the subtree rooted at X

Case 4 - The last step: X is the node we wish to splay

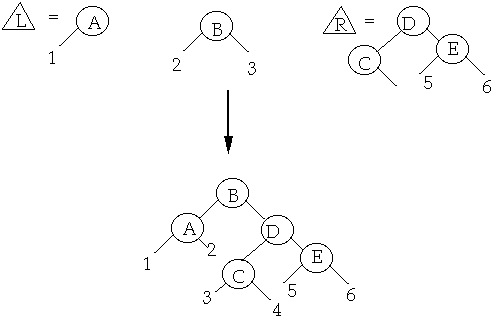
Example Splay at B
 Example Splay at B
Example Splay at B
 CS 660: Combinatorial Algorithms
CS 660: Combinatorial Algorithms CS 660: Combinatorial Algorithms
CS 660: Combinatorial Algorithms